Classifying conformal field theories by means of the Leech lattice

27 June 2024

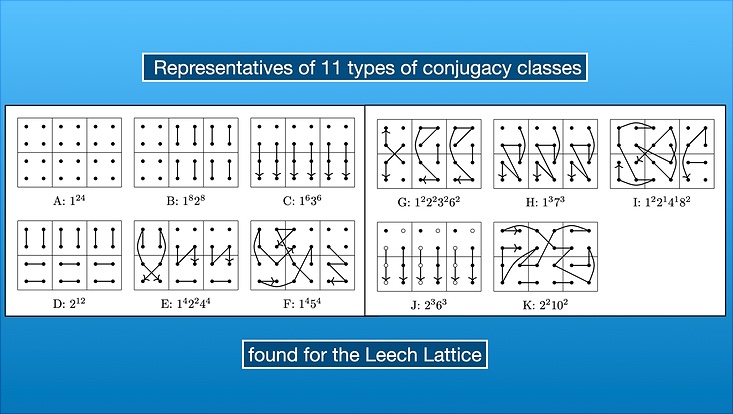
Photo: Möller, S. and Scheithauer, N. (2024)

The diagrams A to K depict the 11 conjugacy classes which the holes of the Leech Lattice project to, as the authors have found, while solving a classification problem in quantum field theory

Mathematician Sven Möller (Universität Hamburg) from the Cluster of Excellence Quantum Universe and his colleague Nils Scheithauer (TU Darmstadt) have developed a beautiful mathematical reduction theory, in a recently published paper. They have used it to solve a long-standing classification problem in quantum field theory, thus providing new insights into the fundamental building blocks of our world.

Quantum field theories (QFTs) are used in particle physics to construct physical models of subatomic particles (such as electrons, quarks or photons). While there is, in general, no rigorous mathematical setting for QFTs yet, some special cases, such as two-dimensional conformal field theories (CFTs), can be formulated rigorously. These CFTs can be axiomatised in the form of vertex operator algebras (VOAs) in mathematics and play an important role in condensed matter physics, statistical mechanics and string theory. On the other hand, VOAs appear in many interesting mathematical problems as well, like Borcherds' Fields medal work, the proof of the monstrous moonshine conjecture.

This recent paper investigates the problem of classifying VOAs, i.e. of answering the question, which VOAs (with certain properties) exist at all, and how they can be distinguished – akin to a biologist categorising all butterfly species with their distinguishing features. In 1993, the physicist Schellekens conjectured that there are exactly 71 VOAs of a certain type (those that are holomorphic and of central charge 24). Since then mathematicians have been trying to solve this problem, developing many powerful tools along the way.

To once and for all solve this taxonomic problem, the authors of the present paper develop a new method to reduce any given VOA of this kind to the Leech lattice VOA. The Leech lattice is in many ways very special and describes the densest sphere packing in 24 dimensions, i.e. the most efficient way to stack 24-dimensional spheres (like a fruit trader stacking oranges in three dimensions). Such lattice models are among the best-understood VOAs and appear prominently in the theory.

What was the key to their reduction method? A generalisation of the concept of ‘holes’ in lattices –  or in the analogy above, essentially the empty spaces between the fruit trader's stacked oranges – which combines ideas from the theory of modular forms and Lie theory. These holes contain a permutation of the 24 basis vectors of the Leech lattice, depicted in the figure above, which groups the holes into 11 subfamilies. Applying this reduction procedure, the authors are able to prove Schellekens' conjecture (with one notable exception, which needs to be addressed with different methods).

The work is a contribution to efforts to ‘chart the landscape’ of 2d CFTs, which themselves are an important piece of the jigsaw in the ongoing quest to understand the mathematical fabric of our universe through quantum field theories and string theory.