Cluster algebras promise simplifications in collider physics calculationsModern mathematical tools may constrain the complexity of Feynman integrals

18. März 2021

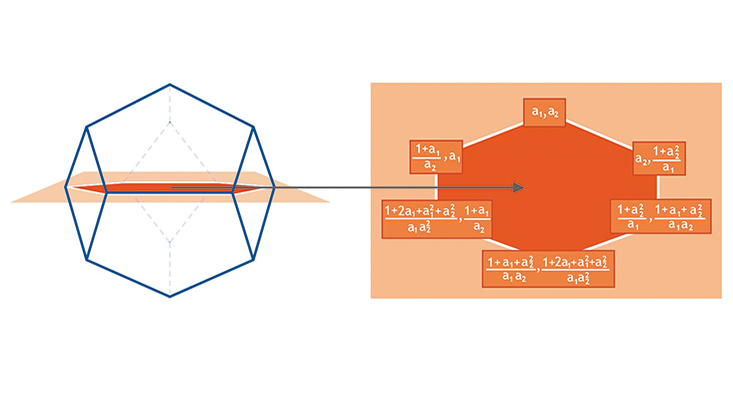
Foto: Max-Planck-Institut für Physik/Carolin Leyck

Theoretical particle physicists compare Feynman integrals to wild beasts that are difficult to tame. In a recent scientific publication, the authors provide evidence that cluster algebras, a new and vibrant area of mathematics, could serve as a principle to control the analytic form of Feynman integrals. This has the potential to simplify theoretical calculations for elementary particle experiments.

To test and to extend the current understanding of Nature, precise theoretical predictions of elementary particle collisions are needed. When the particles interact weakly during the collision, these predictions can be computed by so-called Feynman diagrams. The latter are a method to visualize how the colliding particles will transform to the ones observed after the collision by producing, annihilating and exchanging other unobserved, ’virtual’ particles. As the energy and momentum of these virtual particles may be anything that the laws of Nature allow, one additionally has to integrate over all their possible values. Thus, Feynman diagrams are pictorial representations of exact mathematical expressions known as Feynman integrals. What makes these Feynman integrals a challenge for scientists is that it is in general not known what they evaluate to as functions of the momenta and quantum numbers of the observed particles before and after the collision.

"More efficient predictions of particle collisions in idealized models of quantum theories have been achieved thanks to the beautiful mathematics of cluster algebras, which for that reason have been at the heart of my research for several years. Our current findings open for the first time the exciting prospect of applications of cluster algebras to the real world", says theoretical physicist Georgios Papathanasiou (DESY), key researcher at the Cluster of Excellence Quantum Universe. Together with his colleagues, he discovered that cluster algebras underlie the analytic structure of Feynman integrals contributing to realistic multi-scale processes, such as Higgs production in association with a jet. Cluster algebras are mathematical objects consisting of a set of variables, arranged in overlapping sets known as clusters. In the simplest setting, each cluster can be geometrically understood as a different triangulation of a polygon, with its cluster variables corresponding to the diagonals responsible for this triangulation.    

In the setting of Feynman integrals, cluster variables were found to govern their singularities. This result, which generalizes previous observations about the relevance of cluster algebras in a simplified toy model, may facilitate obtaining results at unprecedented precision: The singularity information they provide has in several cases been essential in determining not only the Feynman integrals, but also the physical quantities they contribute to, via novel bootstrap methods that evade the formidable task of direct integration.